

The last step is to isolate the variable x by itself on the right side of the equation. The opposite of -10 is +10, therefore, we will add both sides by 10.
So, we have to move all the constants to the left side.Ĭlearly, the -10 on the right must be removed. It’s nice to see the variable x just on the right side. After we simplify by adding both sides by 3x, we obtain this less messy equation. To get rid of the -3x on the left side, we add both sides by 3x since the opposite of -3x is +3x. However, for practice, let’s try keeping it on the right side. If you decide to keep the variable on the left side, that is perfectly fine. Now, it is time to decide where to keep the unknown variable x. That is, multiply -2 inside each term in the parenthesis (5-4x). Our very first step should be to get rid of the parenthesis by applying the Distributive Property of Multiplication over Addition. So, we are going to divide both sides by 5, and then we are done! Since +5 is multiplying x, then its opposite operation is to divide by +5. The last step is to isolate the variable x by itself on the left side of the equation. The opposite of +3 is -3, therefore, we will subtract both sides by 3. This implies that we have to move all the constants to the right side by eliminating +3 on the left side. To do that, we need to subtract both sides of the equation by 2x because the opposite of +2x is -2x.Īfter doing so, it is nice to see just the variable x on the left side. This means we will have to get rid of the 2x on the right side. Since 7x is “larger” than 2x, then we might as well keep it to the left side. Notice that there is no parenthesis in this equation and no like terms to combine on both sides of the equation.Ĭlearly, our first step is to decide where to keep or isolate the unknown variable x. This is a typical problem in multi-step equations where there are variables on both sides.
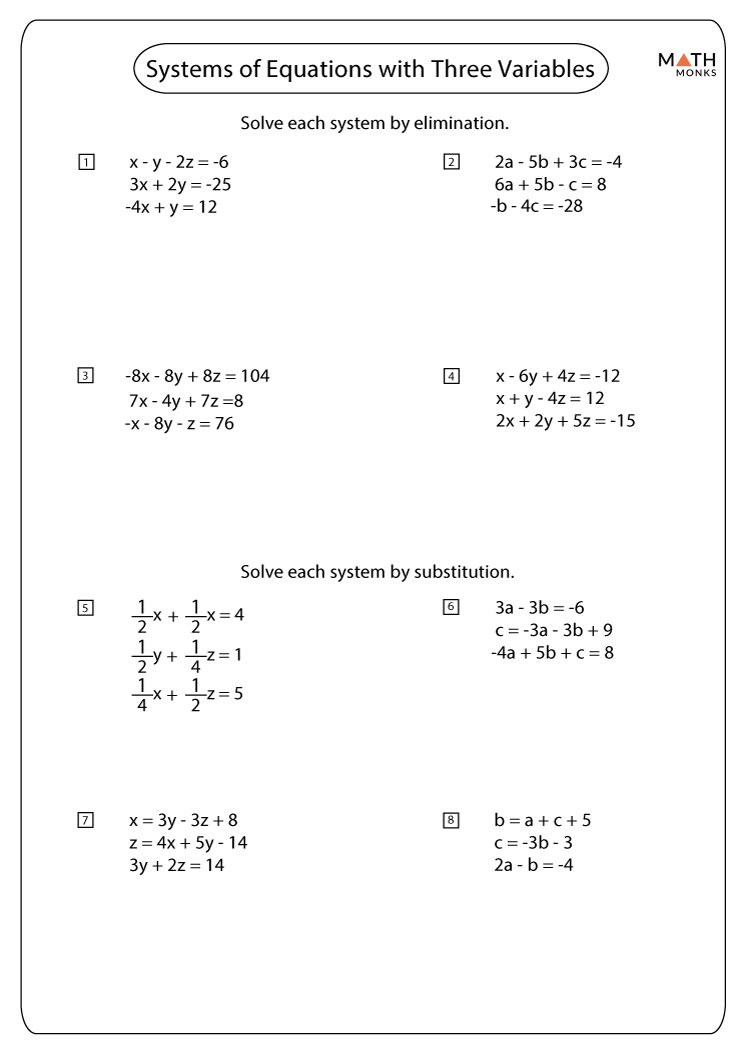
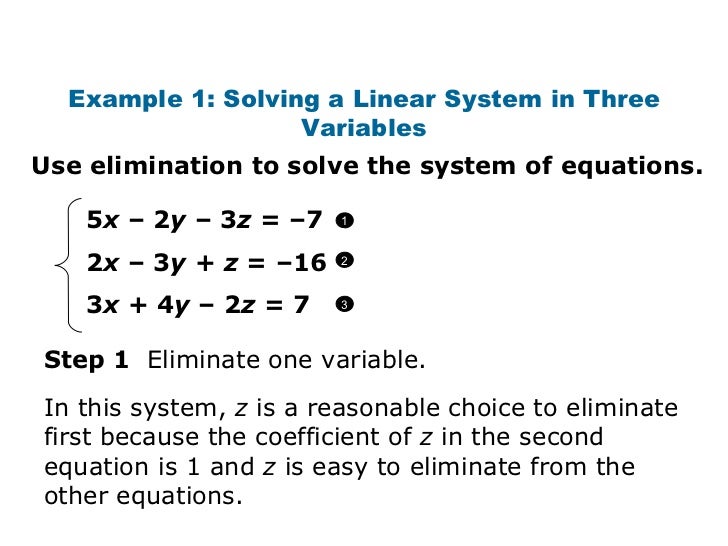
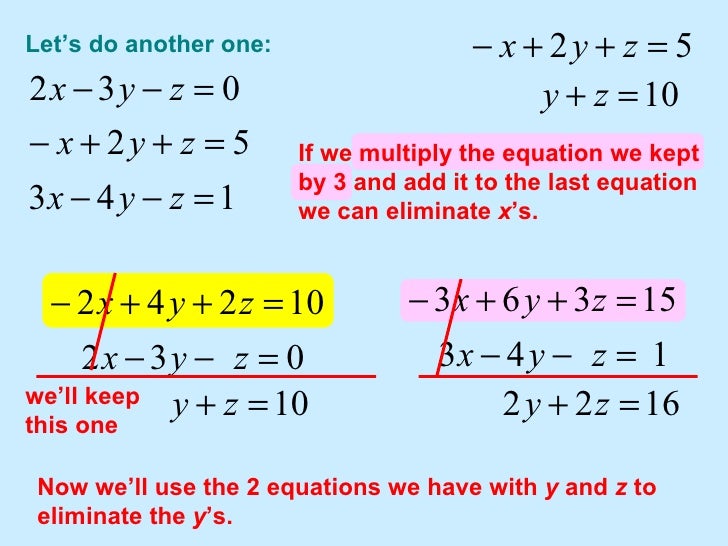
#STEPS FOR 3 EQUATION SYSTEMS HOW TO#
Decide where you want to keep the variable because that will help you decide where to place the constant.Simplify both sides of the equation, if possible, by combining like terms.Get rid of any grouping symbols such as square brackets, parentheses, etc, by applying the Distributive Property of Multiplication over Addition.For example, if we add 5 on the left side of the equation we must also add 5 on the right side.
This concept of performing the same operation on both sides applies to the four arithmetic operations, namely: addition, subtraction, multiplication, and division. Since we are dealing with equations, we need to keep in mind that whatever operation we perform on one side must also be applied on the other to keep the equation “balanced”. But there are cases when it makes more sense to keep it on the right side of the equation. The “standard” or usual way is to have it on the left side. However, there is no rule on where to keep the variable. The main goal in solving multi-step equations, just like in one-step and two-step equations, is to isolate the unknown variable on one side of the equation while keeping the constant or number on the opposite side. That’s why solving multi-step equations are more involved than one-step and two-step equations because they require more steps. The word “multi” means more than two, or many.


 0 kommentar(er)
0 kommentar(er)
